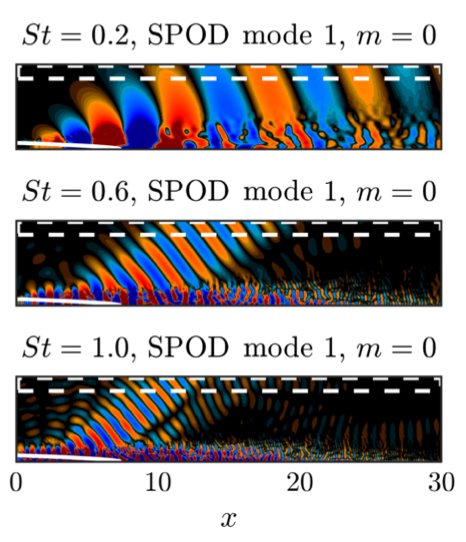
Triadic interactions are the fundamental mechanism of energy transfer in fluid flows. Bispectral mode decomposition (BMD) educes coherent flow structures that are associated with triadic interactions from experimental or numerical data. Triadic interactions are characterized by quadratic phase coupling which can be detected by the bispectrum. The proposed method maximizes an integral measure of this third-order statistic to compute modes associated with frequency triads, as well as a mode bispectrum that identifies resonant three-wave interactions. Unlike the classical bispectrum, the decomposition establishes a causal relationship between the three frequency components of a triad. This permits the distinction of sum- and difference-interactions, and the computation of interaction maps that indicate regions of nonlinear coupling.
Triadic interactions are the fundamental mechanism of energy transfer in fluid flows. Bispectral mode decomposition (BMD) educes coherent flow structures that are associated with triadic interactions from experimental or numerical data. Triadic interactions are characterized by quadratic phase coupling which can be detected by the bispectrum. The proposed method maximizes an integral measure of this third-order statistic to compute modes associated with frequency triads, as well as a mode bispectrum that identifies resonant three-wave interactions. Unlike the classical bispectrum, the decomposition establishes a causal relationship between the three frequency components of a triad. This permits the distinction of sum- and difference-interactions, and the computation of interaction maps that indicate regions of nonlinear coupling.
Literature:
-
![[PDF]](https://flowphysics.ucsd.edu/wp-content/plugins/papercite/img/pdf.png)
![[DOI]](https://flowphysics.ucsd.edu/wp-content/plugins/papercite/img/external.png) Schmidt, O. T.. “Bispectral mode decomposition of nonlinear flows.” Nonlinear dynamics 102(4) (2020): 2479-2501.
Schmidt, O. T.. “Bispectral mode decomposition of nonlinear flows.” Nonlinear dynamics 102(4) (2020): 2479-2501.
[Bibtex] @Article{schmidt_2020_nody,
author = {Schmidt, O. T.},
journal = {Nonlinear Dynamics},
title = {{Bispectral mode decomposition of nonlinear flows}},
year = {2020},
issn = {0924-090X},
number = {102(4)},
pages = {2479-2501},
abstract = {{Triadic interactions are the fundamental mechanism of energy transfer in fluid flows. This work introduces bispectral mode decomposition as a direct means of educing flow structures that are associated with triadic interactions from experimental or numerical data. Triadic interactions are characterized by quadratic phase coupling which can be detected by the bispectrum. The proposed method maximizes an integral measure of this third-order statistic to compute modes associated with frequency triads, as well as a mode bispectrum that identifies resonant three-wave interactions. Unlike the classical bispectrum, the decomposition establishes a causal relationship between the three frequency components of a triad. This permits the distinction of sum- and difference-interactions, and the computation of interaction maps that indicate regions of nonlinear coupling. Three examples highlight different aspects of the method. Cascading triads and their regions of interaction are educed from direct numerical simulation data of laminar cylinder flow. It is further demonstrated that linear instability mechanisms that attain an appreciable amplitude are revealed indirectly by their difference-self-interactions. Applicability to turbulent flows and noise-rejection is demonstrated on particle image velocimetry data of a massively separated wake. The generation of sub- and ultra-harmonics in large eddy simulation data of a transitional jet is explained by extending the method to cross-bispectral information.}},
doi = {10.1007/s11071-020-06037-z},
file = {:Schmidt_2020_NODY.pdf:PDF},
}
Code:
Code and examples from MATLAB Central File Exchange
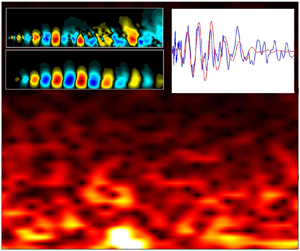 Four different applications of spectral proper orthogonal decomposition (SPOD) are demonstrated on large-eddy simulation data of a turbulent jet. These are: low-rank reconstruction, denoising, frequency–time analysis and prewhitening. We demonstrate SPOD-based flow-field reconstruction using direct inversion of the SPOD algorithm (frequency-domain approach) and propose an alternative approach based on projection of the time series data onto the modes (time-domain approach). We further present a SPOD-based denoising strategy that is based on hard thresholding of the SPOD eigenvalues. The proposed strategy achieves significant noise reduction while facilitating drastic data compression.
Four different applications of spectral proper orthogonal decomposition (SPOD) are demonstrated on large-eddy simulation data of a turbulent jet. These are: low-rank reconstruction, denoising, frequency–time analysis and prewhitening. We demonstrate SPOD-based flow-field reconstruction using direct inversion of the SPOD algorithm (frequency-domain approach) and propose an alternative approach based on projection of the time series data onto the modes (time-domain approach). We further present a SPOD-based denoising strategy that is based on hard thresholding of the SPOD eigenvalues. The proposed strategy achieves significant noise reduction while facilitating drastic data compression.![]()
 The use of spectral proper orthogonal decomposition (SPOD) to construct low-order models for broadband turbulent flows is explored. The choice of SPOD modes as basis vectors is motivated by their optimality and space-time coherence properties for statistically stationary flows. This work follows the modeling paradigm that complex nonlinear fluid dynamics can be approximated as stochastically forced linear systems. The proposed stochastic two-level SPOD-Galerkin model governs a compound state consisting of the modal expansion coefficients and forcing coefficients. In the first level, the modal expansion coefficients are advanced by the forced linearized Navier-Stokes operator under the linear time-invariant assumption. The second level governs the forcing coefficients, which compensate for the offset between the linear approximation and the true state. At this level, least squares regression is used to achieve closure by modeling nonlinear interactions between modes.
The use of spectral proper orthogonal decomposition (SPOD) to construct low-order models for broadband turbulent flows is explored. The choice of SPOD modes as basis vectors is motivated by their optimality and space-time coherence properties for statistically stationary flows. This work follows the modeling paradigm that complex nonlinear fluid dynamics can be approximated as stochastically forced linear systems. The proposed stochastic two-level SPOD-Galerkin model governs a compound state consisting of the modal expansion coefficients and forcing coefficients. In the first level, the modal expansion coefficients are advanced by the forced linearized Navier-Stokes operator under the linear time-invariant assumption. The second level governs the forcing coefficients, which compensate for the offset between the linear approximation and the true state. At this level, least squares regression is used to achieve closure by modeling nonlinear interactions between modes.![[PDF]](https://flowphysics.ucsd.edu/wp-content/plugins/papercite/img/pdf.png)
 Triadic interactions are the fundamental mechanism of energy transfer in fluid flows. Bispectral mode decomposition (BMD) educes coherent flow structures that are associated with triadic interactions from experimental or numerical data. Triadic interactions are characterized by quadratic phase coupling which can be detected by the bispectrum. The proposed method maximizes an integral measure of this third-order statistic to compute modes associated with frequency triads, as well as a mode bispectrum that identifies resonant three-wave interactions. Unlike the classical bispectrum, the decomposition establishes a causal relationship between the three frequency components of a triad. This permits the distinction of sum- and difference-interactions, and the computation of interaction maps that indicate regions of nonlinear coupling.
Triadic interactions are the fundamental mechanism of energy transfer in fluid flows. Bispectral mode decomposition (BMD) educes coherent flow structures that are associated with triadic interactions from experimental or numerical data. Triadic interactions are characterized by quadratic phase coupling which can be detected by the bispectrum. The proposed method maximizes an integral measure of this third-order statistic to compute modes associated with frequency triads, as well as a mode bispectrum that identifies resonant three-wave interactions. Unlike the classical bispectrum, the decomposition establishes a causal relationship between the three frequency components of a triad. This permits the distinction of sum- and difference-interactions, and the computation of interaction maps that indicate regions of nonlinear coupling.
 The mechanics of extreme intensity events in the buffer and logarithmic layers of a turbulent channel at 𝑅𝑒𝜏=2000 is investigated. The 99.9th percentile of the most intense events in the dissipation of turbulent kinetic energy is analysed by means of conditional space–time proper orthogonal decomposition. The computed spatio-temporal modes are coherent in space and over the considered time frame, and optimally capture the energy of the ensemble. The most energetic mode with transverse symmetric structure describes a turbulent burst event. The underlying mechanism is a varicose instability which generates localized extrema in the dissipation and production of turbulent kinetic energy and drives the formation of a hairpin vortex. The most energetic anti-symmetric mode is related to a sinuous-type instability that is situated in the shear layer between two very-large-scale streaks. Statistical results show the energy in the symmetric mode to exceed that in the anti-symmetric mode by a near constant factor for the considered wall distances. Both mechanisms occur throughout the range of wall distances in an effectively self-similar manner that is consistent with the attached-eddy hypothesis. By analogy with transitional flows, the results suggest that the events are induced by an exponential growth mechanism.
The mechanics of extreme intensity events in the buffer and logarithmic layers of a turbulent channel at 𝑅𝑒𝜏=2000 is investigated. The 99.9th percentile of the most intense events in the dissipation of turbulent kinetic energy is analysed by means of conditional space–time proper orthogonal decomposition. The computed spatio-temporal modes are coherent in space and over the considered time frame, and optimally capture the energy of the ensemble. The most energetic mode with transverse symmetric structure describes a turbulent burst event. The underlying mechanism is a varicose instability which generates localized extrema in the dissipation and production of turbulent kinetic energy and drives the formation of a hairpin vortex. The most energetic anti-symmetric mode is related to a sinuous-type instability that is situated in the shear layer between two very-large-scale streaks. Statistical results show the energy in the symmetric mode to exceed that in the anti-symmetric mode by a near constant factor for the considered wall distances. Both mechanisms occur throughout the range of wall distances in an effectively self-similar manner that is consistent with the attached-eddy hypothesis. By analogy with transitional flows, the results suggest that the events are induced by an exponential growth mechanism.